Non-Canonical Partitioning
- Kat Rose

- Mar 24, 2023
- 1 min read
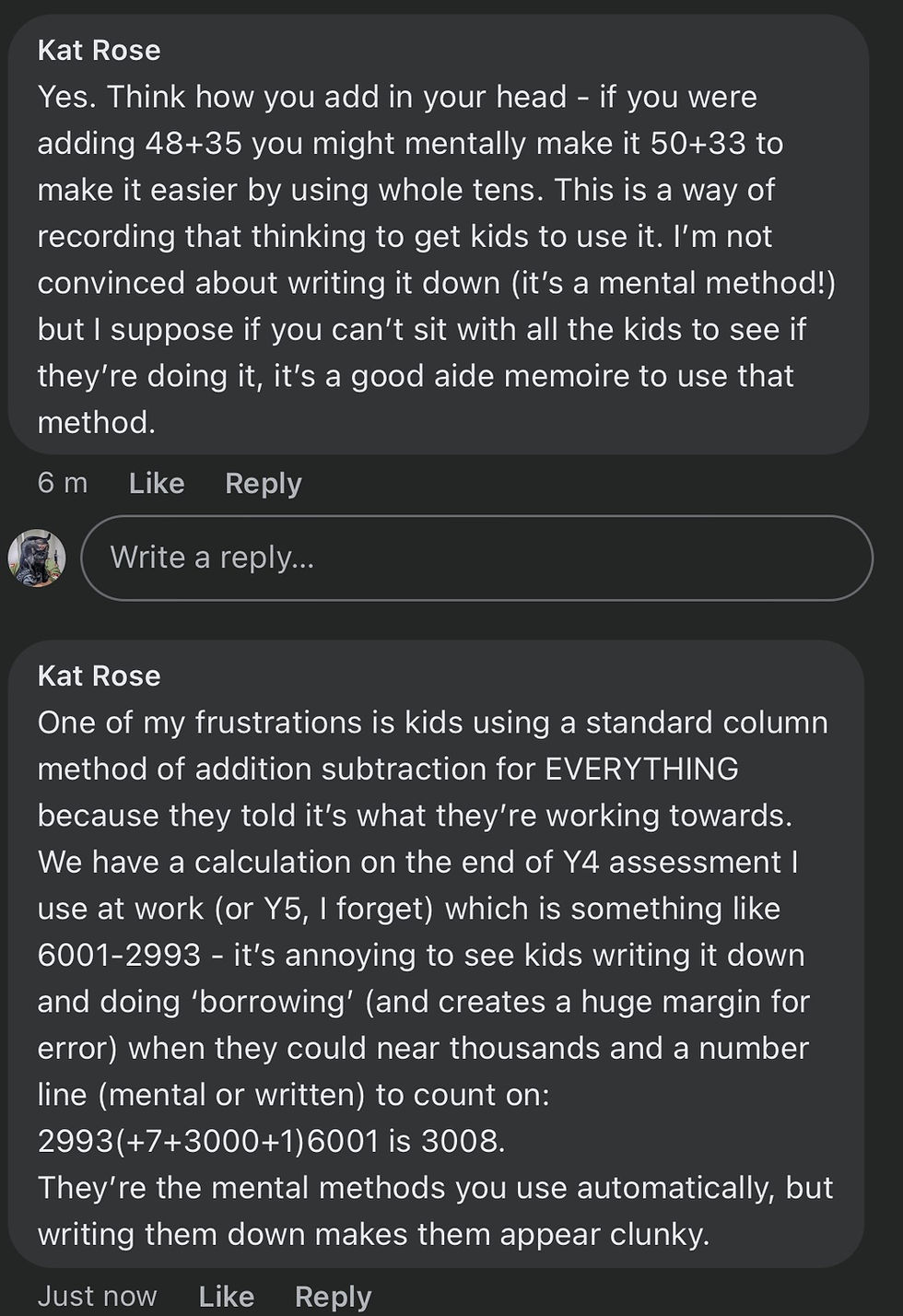
Have you ever heard of ‘non-canonical partitioning’? It’s a big name for ‘splitting up numbers in different ways to make them easier to handle’!
For example, if you are adding nine and two, mentally you might turn them into ten and one to make it easier to cross the tens boundary. And you might not even realise that you do it until someone points it out!

A former teacher of mine shared a piece of homework with a disparaging comment about the method. Whilst I agree that writing it down seems clunky, it’s a written representation of how you might mentally repartition numbers to add them more easily.

And it works for bigger numbers too, as shown in my final comment! (Please forgive the missing words - when I get on my #MathsSpecialistTeacher high horse my brain works faster than my fingers!)




Comments